1. INTRODUCTION The calculation of the geometric properties of a parabolic channel section is accomplished by applying normal and line integrals, making use of the parameters that characterize a parabola.
2. GEOMETRIC PROPERTIES OF A PARABOLIC SECTION
The Manning equation is:
in which Q = flow rate; A = flow area; R = hydraulic radius; So = bottom slope; and n = Manning's roughness. Therefore:
in which the section factor Fs is given as follows:
Therefore:
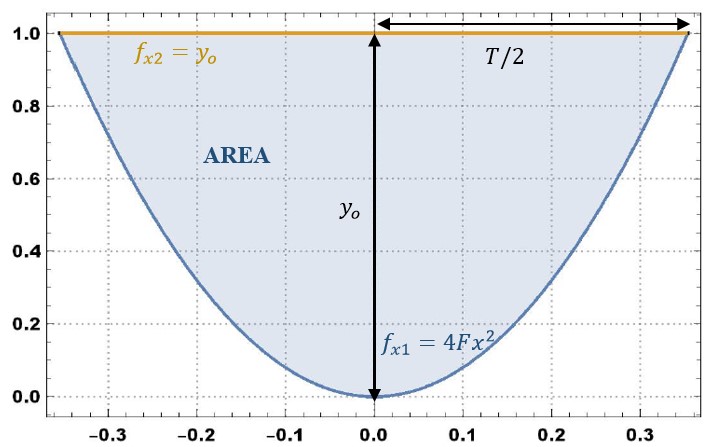
The area and perimeter of a parabolic section corresponding to a flow depth yo and top width T are shown in Fig. 1.
Fig. 1 Definition sketch of a parabolic section. To calculate the area, the following integral is used:
in which 4F is a parameter of the parabola defined as follows:
Therefore:
Substituting Eq. 10 in Eq. 8:
Therefore:
For the wetted perimeter, a line integral is used:
Equation 14 has the form of a known integral: ∫ (a2+x2)1/2dx, and its solution is:
Substituting Eq. 10 in Eq. 16:
Equation 19 may be expressed in terms of logarithms as follows:
Therefore:
Equation 21 is the same formula that appears in Table 2-1 of
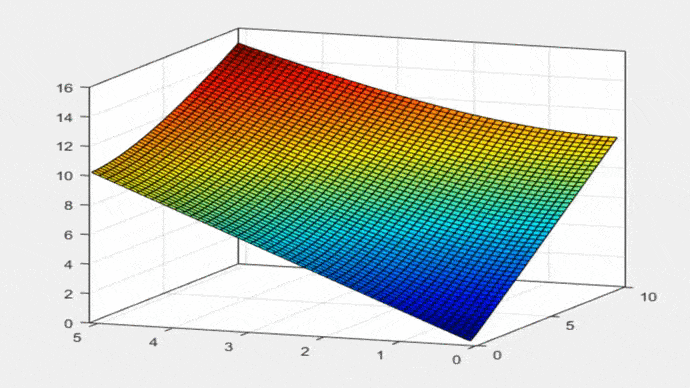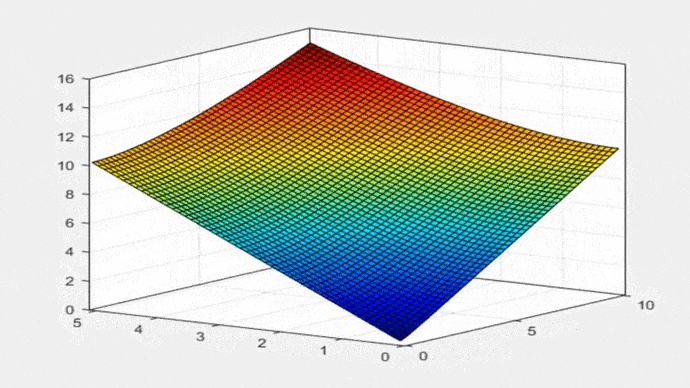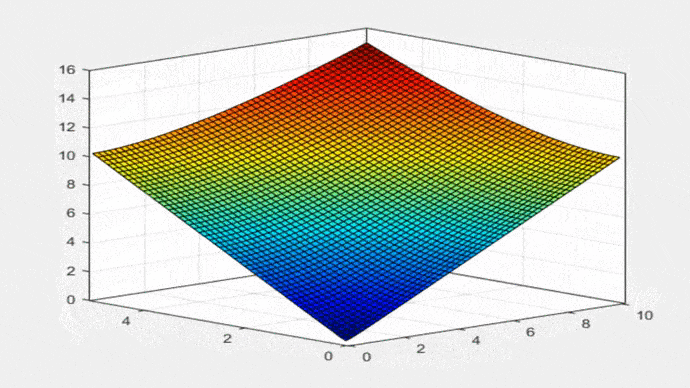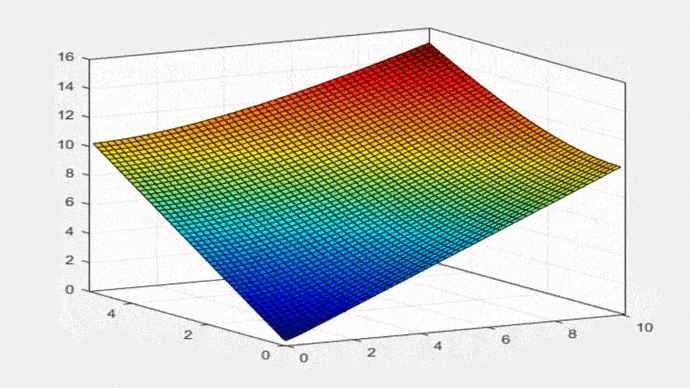
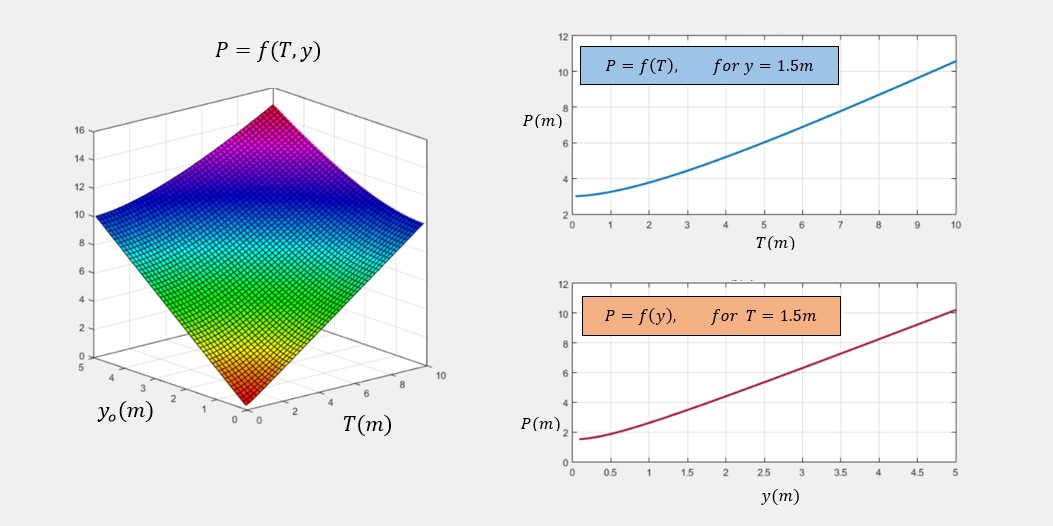
Ven Te Chow's 1959 book: Figure 2 shows Eq. 19 in graphical form: P = f (T,yo), for 0 < T < 10; 0 < yo < 5:
Fig. 2 Wetted perimeter versus flow depth and top width. Equation 19 is used to construct the geometric properties of the parabola. The free surface width T is:
The hydraulic radius R is:
The hydraulic depth D is:
Therefore:
Table 1 summarizes the properties of a parabolic section. The properties may be calculated online using Online geometric elements parabolic.
APPENDIX I. REFERENCES
Chow, V. T. 1959. Open Channel Hydraulics. McGraw-Hill, New York.
Leithold, L. 1998. Calculus, Seventh Edition, Oxford University
Press - Harla México, S.A.
APPENDIX II. NOTATION
The following notation is used in this document:
Q = flow rate;
n = Manning's coefficient;
So = bottom slope;
yo = (center) flow depth;
Fs = section factor;
A = flow area;
P = wetted perimeter;
T = top width;
R = hydraulic radius; and
D = hydraulic depth.
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 210502 04:30 |
| Documents in Portable Document Format (PDF) require Adobe Acrobat Reader 5.0 or higher to view; download Adobe Acrobat Reader. |