1. CONVENTIONAL HYDROLOGIC BALANCE
The conventional hydrologic balance, commonly referred to as the hydrologic budget of a catchment,
is covered in all
standard hydrology textbooks; see, for instance, Ponce (2014).
A hydrologic budget is an accounting of the various transport
phases of the hydrologic cycle within a catchment.
The following is a complete hydrologic budget, including
both surface water and groundwater:
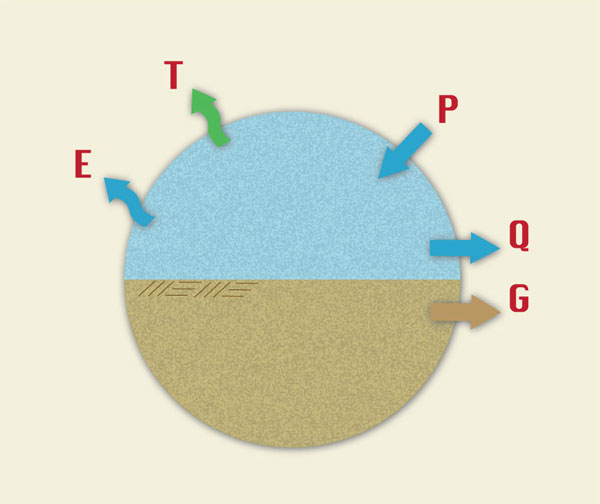
in which ΔS = change in storage, P = precipitation, E = evaporation,
T = evapotranspiration, G = groundwater outflow, and Q = surface runoff.
Equation 1 states that
within a given time span, the change in water volume remaining in storage in a catchment
is the difference between the input (precipitation) and the sum of all outputs
(evaporation, evapotranspiration, groundwater
outflow, and surface runoff).
In hydrologic practice, the terms of Eq. 1 are expressed in units of
water depth, i.e., a water volume uniformly distributed over the catchment area.
Under equilibrium conditions, ΔS = 0, and Eq. 1 reduces to (Fig. 1):
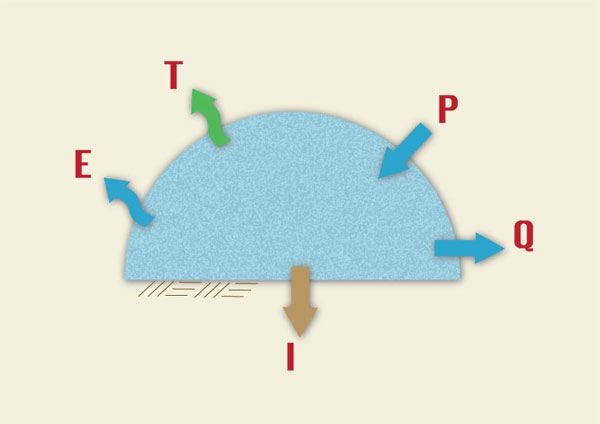
A partial hydrologic budget, which considers only surface water, is:
in which I = infiltration. Within a given time span, under equilibrium conditions
(ΔS = 0),
Eq. 3 reduces to
Equation 4 is imperfect, because it assumes that infiltration amounts are permanently lost to the surface-water budget, which is not quite correct. Within a given time span, say one year, infiltrated amounts may actually return to the control volume (and be eventually released) as evaporation from lakes and ponds, evapotranspiration from vegetation, or as the baseflow component of surface runoff. Notwithstanding its apparent limitations in yield hydrology, Eq. 4 works well in event hydrology (refer to Ponce and Palaniappan, 2014: Facets of Hydrology). In Eq. 4, the hydrologic losses L are defined as the sum of evaporation, evapotranspiration, and infiltration: L = E + T + I. This results in:
which leads to the fundamental equation of flood hydrology:
2. CYBERNETIC HYDROLOGIC BALANCE
On a global annual basis, all precipitation
either: (1) returns to the atmosphere as
vaporization V (through evaporation and evapotranspiration), or
(2) runs off the land as runoff R (i.e., streamflow).
The conventional water balance separates precipitation into: (1) losses,
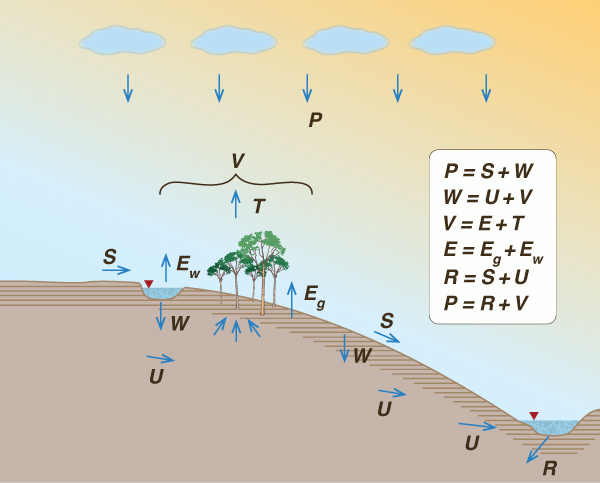
and (2) runoff (Eq. 5). L'vovich (1979) solved this problem by introducing the concept of catchment wetting. He separated annual precipitation into two components (Fig. 3):
in which S = surface runoff, i.e., the fraction of runoff originating on the land surface, and
In turn, wetting is separated into two components:
in which U = baseflow, i.e., the fraction of wetting which exfiltrates as the dry-weather flow of streams and rivers, and V = vaporization, i.e., the fraction of wetting returned to the atmosphere as water vapor. Ostensibly, L'vovich's hydrologic budget neglects deep percolation, the portion of wetting which bypasses the surface waters altogether, estimated at less than 2% of precipitation on a global basis (World Water Balance, 1978). Vaporization, which comprises all moisture returned to the atmosphere, has two components:
in which E = nonproductive evaporation, hereafter referred to as "evaporation," and T = productive evaporation, i.e., that resulting from plant transpiration, hereafter referred to as "evapotranspiration." In turn, evaporation has two components:
in which Eg = evaporation from the ground, bare soil, and small surface storage (puddles), and Ew = evaporation from water bodies such as lakes, reservoirs, streams, and rivers. Evapotranspiration T is the evaporation from vegetated surfaces such as leaves and other parts of plants, a function of the physiological need of plants to pump moisture from the soil to maintain turgor and avail themselves of nutrients.
Runoff (i.e., total runoff) is the sum of surface runoff and baseflow:
Combining Eqs. 7, 8, and 11:
Equations 7 to 12 constitute a set of water balance equations. Combining Eqs. 11 and 12 leads to:
Equation 13 separates annual precipitation into its three major components: (1) surface runoff, (2) baseflow, and (3) vaporization. Significantly, Eq. 13 assumes that the change in soil-moisture storage from year to year is negligible, an assumption which is useful as a first approximation. Under L'vovich's hydrologic budget, two water balance coefficients may be defined: (1) runoff coefficient, and (2) baseflow coefficient. The runoff coefficient is:
The baseflow coefficient is:
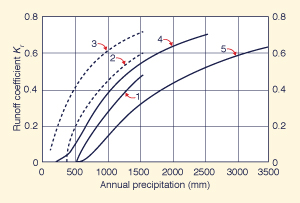
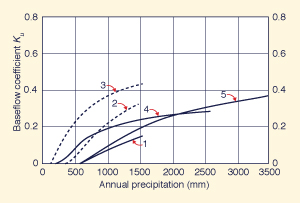
Figure 4 shows runoff and baseflow coefficients calculated by Ponce and Shetty (1995), based on data reported by L'vovich (1979). It is seen that in all cases, runoff and baseflow coefficients increase with annual precipitation.
3. ANALYSIS
It is seen that the conventional hydrologic balance, Eqs. 1 to 6, is deductive and demonstrably based
on a premise of cause-effect. The fundamental approach is subtractive, characterized by
the statement
"runoff is equal to precipitacion minus losses" (Eq. 6).
It works
reasonably well for applications in event hydrology (such as the rational method
of urban hydrology)
but, due to the double counting, it accuracy decreases for the longer-term
yield hydrology.
On the other hand, the cybernetic hydrologic balance, Eqs. 7 to 13, is inductive, not based
on cause-effect but rather on the system's wholeness. The fundamental approach is additive,
characterized by the statement "precipitation is equal to runoff plus vaporization" (Eq. 12). This approach is suited
to applications in yield hydrology, i.e., for determinations of annual baseflow and
runoff coefficients.
4. EXAMPLE APPLICATION
The cybernetic hydrologic balance is applied herein
to data from the
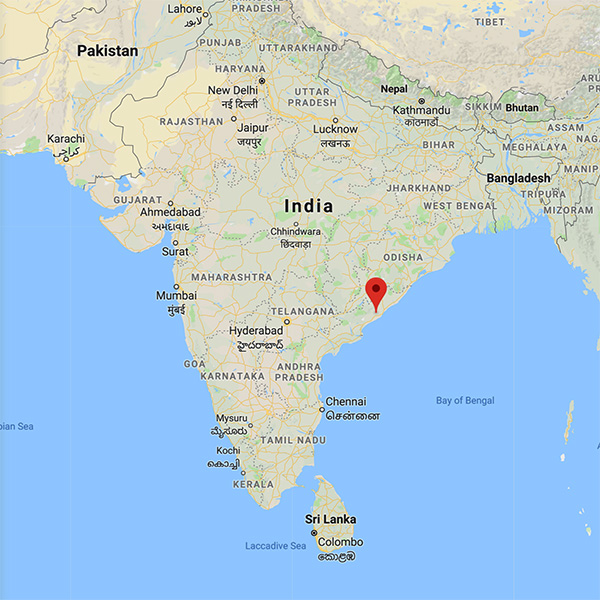
Sarada river basin, upstream of Anakapalli, in Andhra Pradesh, India (Fig. 5).
The basin is positioned between the Eastern Ghats
and the eastern coastline of India, and features a
subhumid climate (Ponce et al., 2000), with a drainage area of 1,980 km2.
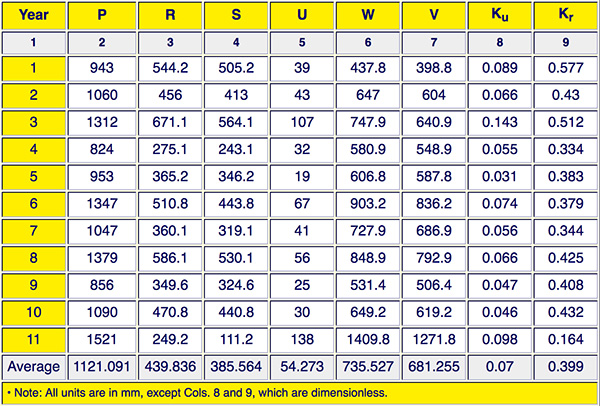
Eleven (11) years of rainfall-runoff data are
analyzed. The precipitation data consists of a daily rainfall hyetograph and
the runoff data consists of the hydrograph measured at the basin outlet.
The annual hyetographs are used to calculate annual rainfall
5. SUMMARY
A comparison between the conventional approach to the hydrologic balance
and L'vovich's catchment wetting approach, referred herein as the
cybernetic approach, reveals fundamental conceptual differences.
The conventional approach is seen to be mostly suited to
event hydrology, particularly for applications of flood hydrology and
urban hydrology.
On the other hand, the cybernetic approach is suited to yield hydrology,
i.e., for determinations of the availability of water resources on an annual basis.
The cybernetic hydrologic balance is applied herein
to data from the
Sarada river basin, upstream of Anakapalli, in Andhra Pradesh, India.
An online calculator
rounds up the experience (Online water balance).
References
L'vovich, M. I. 1979. World water resources and their future.
Translation from Russian by Raymond L. Nace, American Geophysical Union.
Ponce, V. M., and A. V. Shetty. 1995.
A conceptual model of catchment balance: 2. Application to runoff and baseflow modeling. Journal of Hydrology, 173, 41-50.
Ponce, V. M., R. P. Pandey, and S. Ercan. 2000. Characterization of drought across climatic spectrum.
Journal of Hydrologic Engineering, ASCE, Vol. 5, No. 2, April, 222-224.
Ponce, V. M. 2014. Engineering hydrology: Principles and practices.
Online text; Second edition.
World water balance and water resources of the Earth. (1978). USSR Committee for the International Hydrologic Decade, UNESCO, Paris.
|
| 220824 11:00 |